Download PDF
Download page Workshop 1 – Simple Yield Analysis.
Workshop 1 – Simple Yield Analysis
Fact Sheet
LAKE TED HILLYER
Reservoir Information
Location: On the Purple River above the confluence with the Orange River.
Purpose: Water Supply, Flood Control
Lake Data: Based on current sedimentation survey
sFeature | Elevation (feet) | Area (acres) | Capacity (acre-feet) |
Top of Dam | 1095.0 | 53,300 | 3,070,000 |
Top of Flood Control Pool | 1085.0 | 47,182 | 2,554,000 |
Top of Conservation Pool | 1072.0 | 39,078 | 1,994,000 |
Top of Buffer Pool | 1054.0 | 30,587 | 1,370,000 |
Bottom of Conservation Pool | 1035.0 | 22,442 | 867,000 |
Operating Zone | Capacity (acre-feet) |
Flood Control | 560,000 |
Conservation (total) | 1,127,000 |
Buffer (included in Conservation Zone) | 503,000 |
Inactive | 867,000 |
Model Information
Networks
- Basic Network – Contains a single reservoir (Upper)
Operations Sets
- Basic Min Release – Simple minimum release
Rules
- Min Release – Specifies a constant release year-round in all zones for water supply
Alternatives
- Basic – Standard run with inflow time series only
Simulations
- Full Period – Runs the full historical period (1943 – 1993)
Workshop #1 – Simple Yield Analysis
Part 1.a – Sequent Peak Method
- Open the Excel spreadsheet saved as …\Workshop1\Workshop #1.xlsx
- This spreadsheet contains a pre-populated example of the sequent peak method calculations and plots on the "Sequent Peak Method" sheet.
- The monthly average inflow to a reservoir is stored in column B.
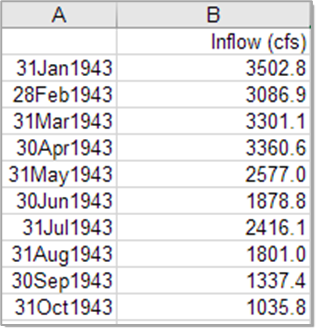
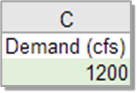
- A hypothetical demand for water supply is defined in Cell C2. This demand is applied to every month in the period of record (1943 – 1993).
- Columns D and E convert the inflow and demand from flow (cfs) to volume (1000 acre-feet [KAF]).
- Columns F and G calculate the accumulated net inflow each month. The net inflow is defined as the gross inflow (column D) minus the demand (column E).
- Columns H and I calculate the accumulated shortage each month. Shortage occurs when the inflow in a month is less than the demand. The accumulated shortage can never be less than zero. If the inflow is larger than the demand for multiple months in a row, the reservoir has surplus water, and the shortage is set to zero.
- The chart to the right of the data table shows the two accumulated time series together, Net Inflow (blue) on the left axis, and Shortage (pink) on the right.
- The storage required to meet the input demand can be determined in two ways:
- The accumulated net inflow time series (blue) can be examined to find the largest drop between a peak and the subsequent trough. The difference between the peak and trough is the required storage.
- The accumulated shortage can also be used. The largest accumulated shortage across the full period is the required storage.
- The results of these two methods are the same.
- Play with the demand by changing the value in Cell C2. See what happens on the plot when the demand changes. Pay attention to the scales on both vertical axes, they will change to fit the data as the demand changes.
- What happens if the demand decreases? Does the required storage increase or decrease? How much? Is the critical period the same?
The required storage decreases as demand decreases. See table for various levels of demand. The critical period switches from the 1950's to 1986 for demand less than 715.
Demand (cfs) | Required Storage (KAF) | Critical Period |
233 | 0 | – |
600 | 45 | 1986 |
715 | 71 | 1950's / 1986 * |
900 | 149 | 1950's |
1000 | 229 | 1950's |
*The 1950s and 1986 droughts are equally critical at a demand of about 715 cfs.
- What happens if the demand increases? Does the required storage increase or decrease? How much? Is the critical period the same? What is the upper bound on demand?
The required storage increases. See table for various levels of demand. The critical period switches from the 1950's back to the 1980s above demand of 1515 cfs.
Demand (cfs) | Required Storage (KAF) | Critical Period |
1200 | 454 | 1950's |
1515 | 1054 | 1950s / 1980s * |
1800 | 1953 | 1980s |
1893 | 2388 *** | 1980s |
2081 | 3569 ** | 1980s |
*The 1950s and 1988 droughts are equally critical at a demand of about 1515 cfs.
**The upper bound on demand is the average inflow of 2081 cfs, which requires storage of 3569. However, this demand ignores the fact that the accumulated net inflow is insufficient to provide that demand, and the critical period does not show recovery to zero by the end of the record.
***The highest demand that doesn't cause a negative net inflow and allows recovery of the accumulated shortage to zero is 1890 cfs.
- What happens if the demand is set to a higher flow than the long-term average inflow (2081 cfs)? Can this demand be met? What happens to the accumulated net inflow? Are the results of this calculation still valid in this scenario?
The demand cannot be met, there is not enough inflow regardless of reservoir size. The accumulated net inflow becomes negative. The sequent peak method has no result in this range.
- Results
- Determine the required storage for a demand of 1000 cfs. What is the critical period for this demand?
229 KAF. Critical period is August 1955 – December 1957.
- Determine the required storage for a demand of 1800 cfs. What is the critical period for this demand?
1953 KAF. Critical period is August 1984 – December 1992.
Part 1.b – Rippl Method (time permitting)
- In the same spreadsheet, the "Rippl Method" sheet contains an example of the same analysis done using the Ripple Method.
- The plot shows the accumulated inflow (note this is now the total inflow, not net inflow as in part 1) and a demand line with a slope matching the demand input in Cell C2. The plot is zoomed into the first drought in the period.
- The required storage to meet the demand is found as the largest vertical distance between the accumulated inflow and the demand line.
- The starting date for the demand line may need to be changed to ensure it is tangent to the accumulated inflow at the beginning of the critical period. You can change the date in Cell G6 to set where the demand line begins.
- Try some of the same demands you used in part 1 of this workshop. Change the start date for the demand line accordingly, and try to measure the required storage as the maximum distance between the two lines (the demand line should be above the inflow line). Do you get the same results?
Yes, the results are the same, though it can be hard to accurately calculate storage sizes visually especially for smaller demands.
Part 2 – Iterative Simulation
- Open the HEC-ResSim watershed stored in …\Workshop1\Workshop1.wksp
- Go to the Watershed Setup Ensure that the ‘One Res’ configuration is selected.

- Examine the layout of this watershed. There are two rivers and (for now) a single reservoir. There are a number of computation points downstream of the reservoir. This basic layout will be used for all of the HEC-ResSim tasks in this course.
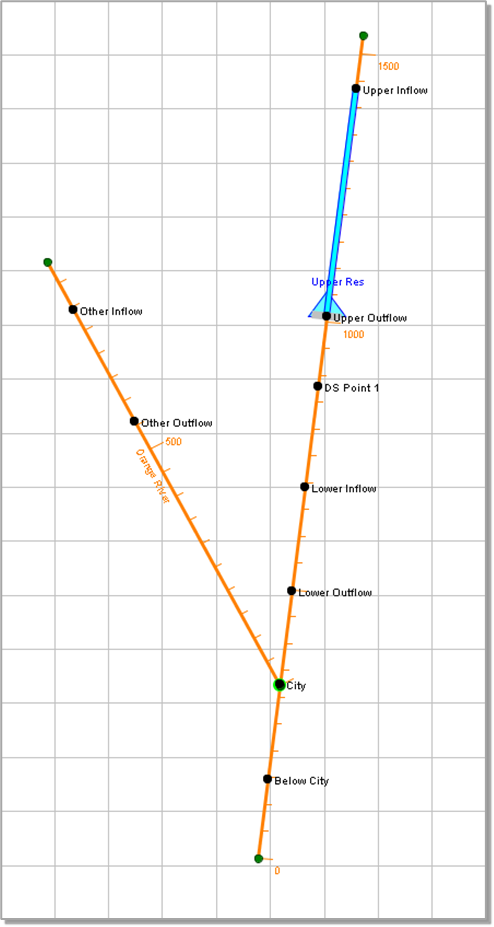
- Go to the Reservoir Network Open the ‘Basic Network’ network.
Notice that the computation points downstream of the reservoir are not included in this network. They are not needed yet.
- Open the Reservoir Editor for ‘Upper Res’ by double clicking on the reservoir. Go to the Physical tab.
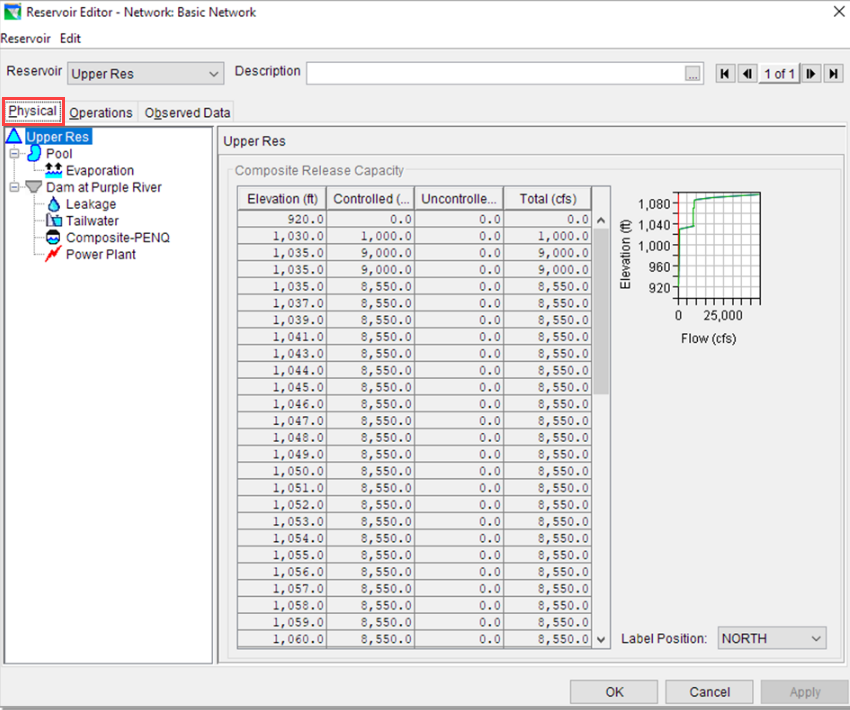
- Examine all the physical components of this reservoir.
- Look at the elevation-storage-area relationships under Pool. How much water can this reservoir hold? How big is the water surface when completely full?
Maximum storage is 3.07 MAF. Maximum surface area is 53,300 acres.
- Look at the Evaporation. This workshop is setup with zero evaporation each month. Think about how the yield and required storage might change if evaporation is included.
NOTE: Evaporation is a critical input to a water supply study and can have significant effects on the result. We are ignoring evaporation for this workshop to focus on the mechanics of firm yield analysis but will include evaporation in later workshops.
Yield will decrease for the same storage, or storage needs will increase for the same yield.
- There is a Leakage component on the dam, but the leakage is zero for this workshop.
- Tailwater stage is defined using a simple rating curve. Tailwater stage is used in calculating hydropower production.
- There is a single controlled outlet named Composite-PENQ. This outlet represents the composite capacity of all available non-turbine outlets on the reservoir. For our modeling, there is no need to specify individual outlet capacities. Some studies may require this.
- A Power Plant is also defined. The outlet capacity is dependent on the reservoir elevation and is not a monotonic function. Capacity decreases at high heads, as is common with turbines.
- Go to the Operations tab. We have one operation set defined so far. The reservoir is split into 5 elevation zones, each with a constant year-round top elevation. The zones are shown graphically on the right side of the tab. In the list on the left side of the tab, Conservation and Inactive zones are formatted differently than the rest. HEC-ResSim considers these zones special.
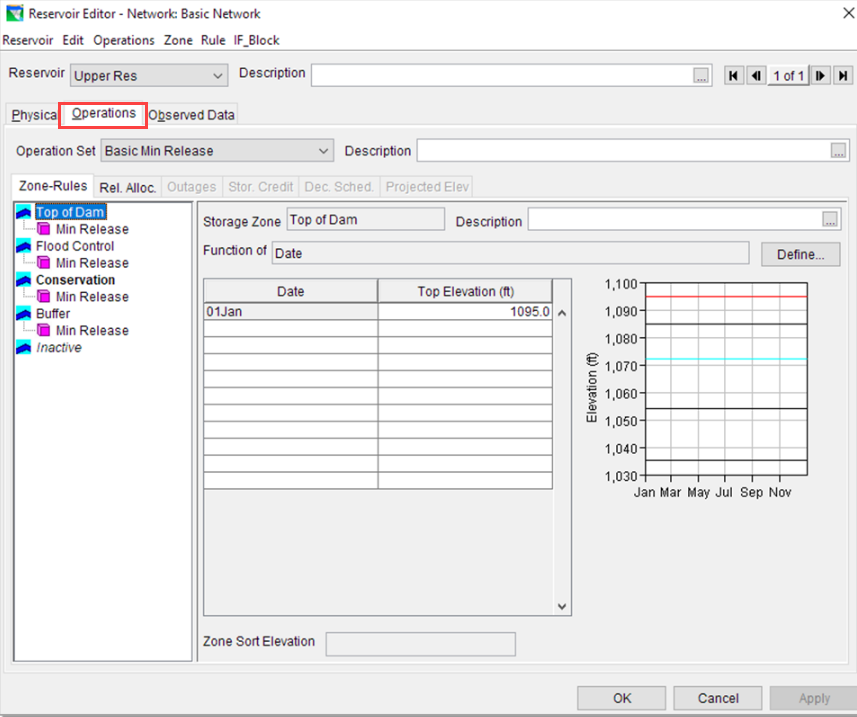
- The Conservation zone is bold, indicating it is set as the Guide Curve for the reservoir.
- The model will attempt to keep the simulated storage equal to the guide curve at all times, unless a rule or physical capacity limit prevents it.
- The Conservation zone is bold, indicating it is set as the Guide Curve for the reservoir.
- The Inactive zone is italic, indicating it is set as the Inactive pool for the reservoir.
- The Inactive zone cannot contain rules, as no operations are possible when the reservoir is that low.
- The Inactive zone is italic, indicating it is set as the Inactive pool for the reservoir.
![]()
- There is one rule in each zone, except for the Inactive zone. The same rule is used in each zone, and changes made to one copy will apply to all the others. The ‘Min Release’ rule specifies a minimum release in each timestep. This rule corresponds to the demand from the spreadsheet example. The initial demand value is 1000 cfs.
- Close the Reservoir Editor.
- Open the Junction Editor and look at the ‘Upper Inflow’ junction.
Note that there is a local inflow defined at this junction with the name “Upper Inflow LOC”.
- Close the Junction Editor.
- Open the Alternative Editor.
- Select the ‘One Res’ configuration and the ‘Basic Network’ alternative.
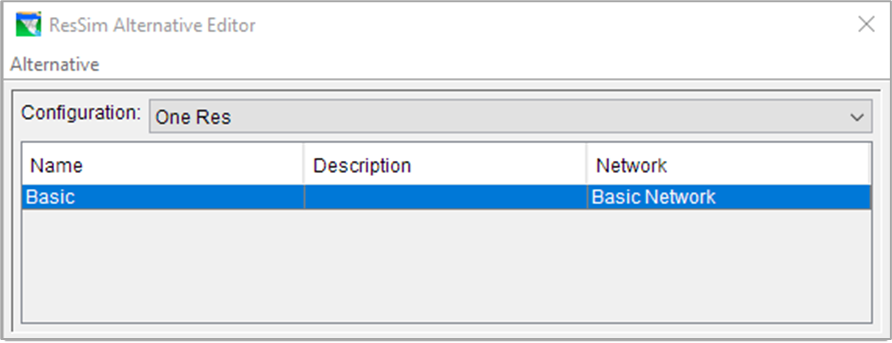
- On the Run Control tab, see the simulation timestep and the Alternative Type. While many water supply studies may only require monthly analysis, HEC-ResSim runs at daily or smaller timesteps. Daily is short enough for this study. Make sure the Alternative Type is set to Standard, for now. We will explore the Yield Analysis type later. Make sure that the Compute Unregulated Flows box is checked and the Compute Holdouts box is unchecked. Set the Log Level to 3.
- On the Operations tab, note that the operations set we looked at earlier is selected.
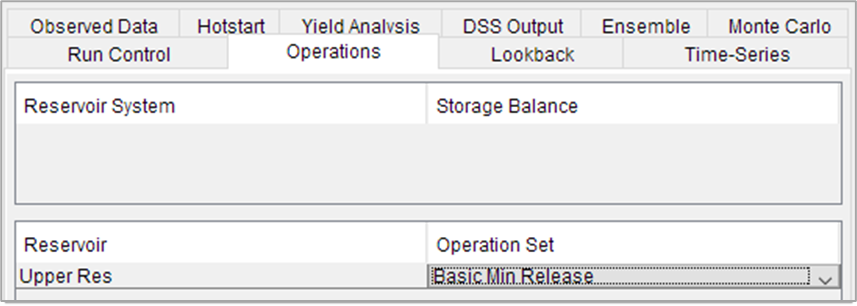
- The Lookback tab specifies starting values for different model time series. These values are not critical, as long as they are set to a reasonable value. If in doubt, set the Lookback Elevation to the guide curve, and the Lookback Releases to zero.
- The Time Series tab maps the needed inputs to time series saved in a separate HEC-DSS file. Our simple model has only one input for now – the inflow at the upstream end of the reservoir. This is the same inflow we saw in the sequent peak spreadsheet.
- Close the Alternative Editor.
- Go to the Simulation Module.
- Open the ‘Full Period’ simulation.

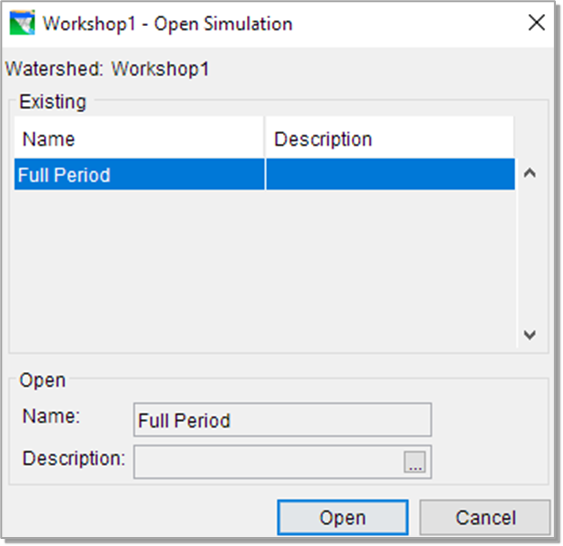
- Note the period of record for this simulation. Note the one included Alternative. Make sure the ‘Basic’ alternative is active and checked.
- Run the simulation.

- Several built-in plots are available by right clicking on the reservoir. The Plot Operations plot will be useful for this workshop, as it shows storage by default. Feel free to look at the other plots as well.
- Open the Plot Operations plot. Note the storage on the top subplot. How low does it get with the initial demand of 1000 cfs? Is it close to the bottom of the conservation zone (which is the top of the inactive zone)?
The minimum storage is 1,761,000 ac-ft. It is not close to the bottom of the conservation zone.
- Note how the minimum release of 1,000 cfs from our rule prevents the model from releasing less than that value at any point in the record. Note how the physical outlet capacities we defined also limit the release, and force the reservoir to store water in the flood control zone occasionally.
- How does the size of the conservation zone (reference the project fact sheet) compare to the required storages we saw in part 1?
The conservation zone extends from elevation 1035 ft (867,000 ac-ft) to 1072 ft (1,994,000 ac-ft). This is a volume of 1,127 KAF. This volume is in between the required storage for the 1515 cfs and 1800 cfs demands from part 1.
- Change the minimum release to 1800 cfs by right-clicking the reservoir and selecting Edit Reservoir Properties. Change the value in the minimum release rule, then click OK or Apply to save your changes. Re-run the simulation. What happens to the simulated storage? Are we able to meet this demand with the available storage?
The simulated storage drops further and more frequently. On several occasions it reaches and stays at the bottom of the conservation pool. We cannot meet the demand with this storage, as shown by the simulated release dropping below the 1800 cfs minimum when the resevoir empties. This matches part 1, which determined a storage of 1,953 KAF was needed to provide 1800 cfs of firm yield – larger than the conservation pool.
- Try to figure out the firm yield of the conservation pool as it is currently modeled. Change the minimum release until the release is able to be met at all times, while drafting the simulated storage down to the bottom of the conservation pool. The Plot Operations plot can be used to visualize the results to help with this task. Also, the Reservoir Summary Report can be used to see the minimum pool elevation and storage during the run.
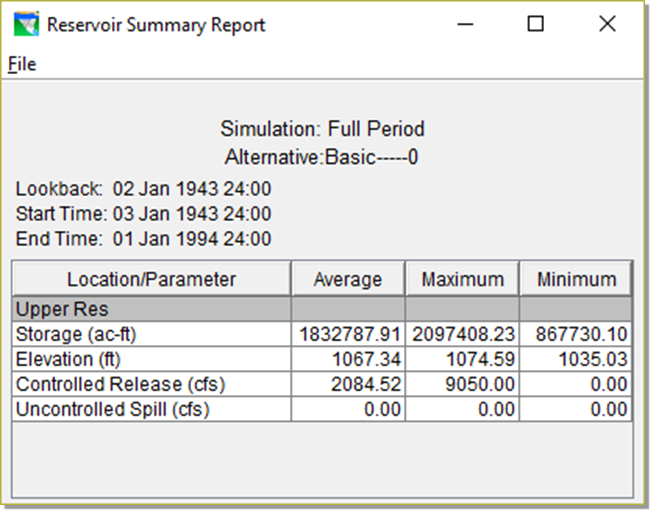
The yield of this model is 1535 cfs, though student answers may vary slightly depending on how precise they try to be.
- What is the actual minimum release during your full period of record? Is it the same as the minimum release you specified in your rule?
The actual minimum release achieved during the simulation will vary depending on the release specified in the ‘Min Release’ rule. The minimum value from the simulation should be close to the value set in the rule but may be a bit lower if the value in the rule is a little above the actual firm yield amount (causing a failure to meet the release in at least one period). The result of using 1535 cfs in the rule is a minimum simulation release of 1535 cfs.
- What is the minimum storage reached in the period? How close it is to the bottom of the conservation pool?
The actual minimum storage will vary depending on the exact minimum release used. It should be close to the bottom of the conservation pool. The result of using 1535 cfs is a minimum storage of 867,730 ac-ft.
- What is the critical period for this reservoir?
June 1954 – February 1961.
- Go back to your spreadsheet from Part 1 of this workshop. Use the firm yield you found in step 20 above as the demand in the spreadsheet. What required storage do you get? Does it match the size of the conservation zone in the HEC-ResSim model? Why or why not?
Using a demand of 1535 cfs gives a required storage (maximum accumulated shortage) of 1,117 KAF. This is slightly smaller than the conservation zone size of 1,127 KAF. These differences are likely due to the difference between daily HEC-ResSim modeling and monthly sequent peak analysis, but overall, for this very simple model, the iterative model simulation approach gives a very similar result to the sequent peak method.